Have you ever wondered how a seemingly simple line can fill an entire space? This captivating video embarks on a journey to explore the fascinating world of space-filling curves, using everyday objects like water, mazes, and even 3D printed models to bring these mathematical concepts to life.
The Intriguing Hilbert Curve: Water Reveals Its Secrets

The journey begins with a familiar sight: water flowing through a maze. But instead of the traditional path, the water takes a surprising turn, mimicking the intricate twists and turns of a Hilbert curve, a type of space-filling curve. While this isn’t a perfect example due to the limited iterations, it sparks the question: how can a one-dimensional curve fill a two-dimensional space?
The video delves deeper, explaining that space-filling curves are fractals, meaning they exhibit self-similarity and infinite detail. Imagine zooming into the Koch snowflake, a classic fractal; you’ll see smaller versions of the same shape endlessly repeating. Space-filling curves work similarly, their infinitely thin lines weaving intricate patterns to fill the entire space.
Beyond Theory: Visualizing Space-Filling Curves in Unexpected Ways
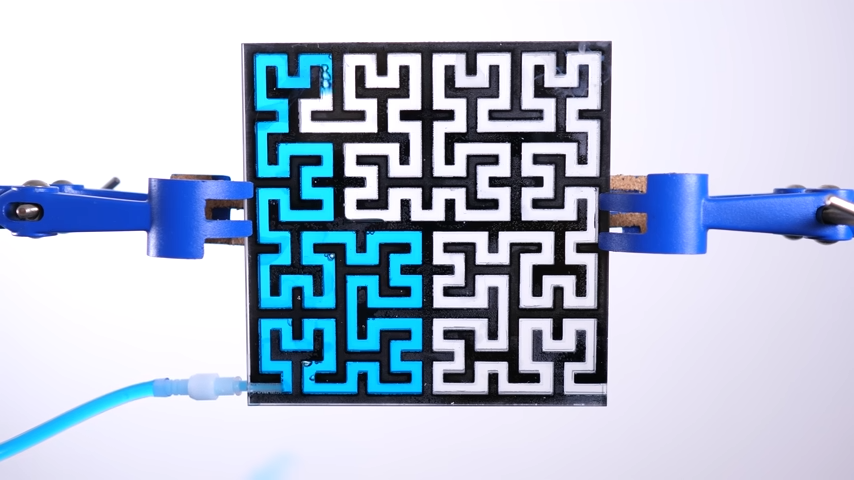
But how does this translate from theory to reality? The video showcases various ways to visualize these curves:
- Water filling a 2D Hilbert curve: Watching the water gradually fill the intricate path provides an intuitive understanding of the curve’s structure.
- 3D printing a Hilbert curve: This tangible model allows for stretching and manipulating the curve, demonstrating its unique properties.
- Marble run inspired by the Hilbert curve: The marbles navigate the intricate pathways, offering a dynamic and visually appealing representation.
Open Questions and Mathematical Challenges: Can We Prove Everything?
The video also explores the limitations of current mathematical tools in proving that certain curves, like the Celtic labyrinth, are truly space-filling. This open question highlights the ongoing development of mathematics and the human quest to understand the infinite.
Inspiration Strikes: A Novel Marble Run Unveils the Hilbert Curve
Inspired by AlphaPhoenix’s work, the video presents a novel way to visualize the Hilbert curve: a 3D printed marble run. As the marbles travel through the intricate loops, the analogy to an electric circuit adds another layer of understanding.
Unveiling the 3D Hilbert Curve: A Looping Marvel with Hidden Depths

Finally, the video showcases a 3D printed version of the Hilbert curve, highlighting its fascinating properties like its “loopiness” and ability to be stretched. By manipulating the surface with a refractive liquid, the video enhances its clarity and visual appeal.
A Journey of Curiosity and Discovery: Beyond the Lines
Throughout the exploration, the video maintains a lighthearted and engaging tone, inviting viewers to participate in the discovery process. It encourages curiosity, challenges our understanding of space and dimension, and leaves us marveling at the beauty and complexity of mathematical concepts.
So, the next time you see a maze or a winding path, remember the hidden world of space-filling curves, where lines dance and defy our expectations, reminding us that the universe is full of fascinating surprises waiting to be explored.